3 Liquid-liquid Extraction
Staged Liquid-Liquid Extraction and Hunter Nash Method
![]() = extract leaving stage
= extract leaving stage ![]() . This could refer to the mass of the stream or the composition of the stream.
. This could refer to the mass of the stream or the composition of the stream.
![]() = solvent entering extractor stage 1. This could refer to the mass of the stream or the composition of the stream.
= solvent entering extractor stage 1. This could refer to the mass of the stream or the composition of the stream.
![]() = generic stage number
= generic stage number
![]() = Final stage. This is where the fresh solvent S enters the system and the final raffinate
= Final stage. This is where the fresh solvent S enters the system and the final raffinate ![]() leaves the system.
leaves the system.
![]() = Composition of the mixture representing the overall system. Points (
= Composition of the mixture representing the overall system. Points (![]() and
and ![]() ) and (
) and (![]() and
and ![]() ) must be connected by a straight line that passes through point
) must be connected by a straight line that passes through point ![]() .
. ![]() will be located within the ternary phase diagram.
will be located within the ternary phase diagram.
![]() = Operating point.
= Operating point. ![]() is determined by the intersection of the straight line connecting points (
is determined by the intersection of the straight line connecting points (![]() ,
, ![]() ) and the straight line connecting points (
) and the straight line connecting points (![]() ,
, ![]() ). Every pair of passing streams must be connected by a straight line that passes through point
). Every pair of passing streams must be connected by a straight line that passes through point ![]() .
. ![]() is expected to be located outside of the ternary phase diagram.
is expected to be located outside of the ternary phase diagram.
![]() = raffinate leaving stage
= raffinate leaving stage ![]() . This could refer to the mass of the stream or the composition of the stream.
. This could refer to the mass of the stream or the composition of the stream.
![]() = solvent entering extractor stage
= solvent entering extractor stage ![]() . This could refer to the mass of the stream or the composition of the stream.
. This could refer to the mass of the stream or the composition of the stream.
![]() = mass ratio of solvent to feed
= mass ratio of solvent to feed
![]() = Mass fraction of species
= Mass fraction of species ![]() in the raffinate leaving stage
in the raffinate leaving stage ![]()
![]() = Mass fraction of species
= Mass fraction of species ![]() in the extract leaving stage
in the extract leaving stage ![]()
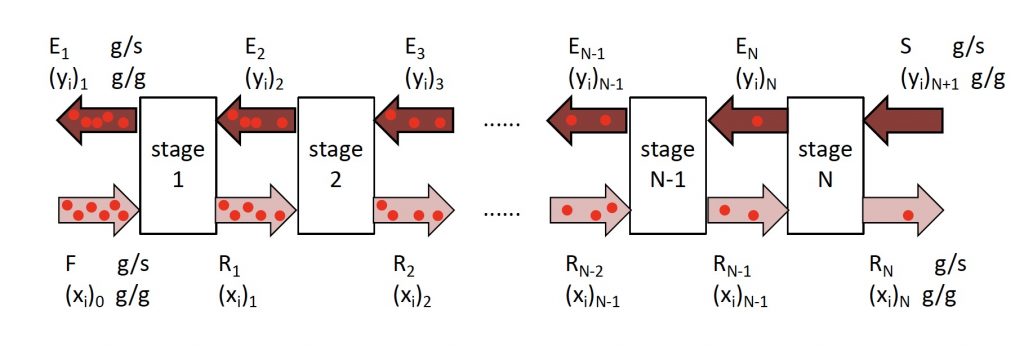
Determining number of stages ![]() when (1) feed rate; (2) feed composition; (3) incoming solvent rate; (4) incoming solvent composition; and (5) outgoing raffinate composition have been specified/selected.
when (1) feed rate; (2) feed composition; (3) incoming solvent rate; (4) incoming solvent composition; and (5) outgoing raffinate composition have been specified/selected.
- Locate points
 and
and  on the ternary phase diagram. Connect with a straight line.
on the ternary phase diagram. Connect with a straight line. - Do a material balance to find the composition of one species in the overall mixture. Use this composition to locate point
 along the straight line connection points
along the straight line connection points 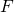 and
and 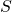 . Note the position of point
. Note the position of point 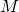 .
. - Locate point
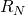 on the ternary phase diagram. It will be on the equilibrium curve. Draw a straight line from
on the ternary phase diagram. It will be on the equilibrium curve. Draw a straight line from 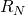 to
to 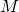 and extend to find the location of
and extend to find the location of 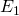 on the equilibrium curve.
on the equilibrium curve. - On a fresh copy of the graph, with plenty of blank space on each side of the diagram, note the location of points
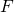 ,
, 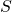 , and
, and 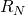 (specified/selected) and
(specified/selected) and 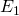 (determined in step 3).
(determined in step 3). - Draw a straight line between
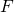 and
and 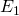 . Extend to both sides of the diagram. Draw a second straight line between
. Extend to both sides of the diagram. Draw a second straight line between 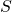 and
and 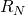 . Note the intersection of these two lines and label as “
. Note the intersection of these two lines and label as “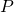 ”.
”. - Determine the number of equilibrium stages required to achieve the desired separation with the selected solvent mass.
– Stream ![]() is in equilibrium with stream
is in equilibrium with stream ![]() . Follow the tie-lines from point
. Follow the tie-lines from point ![]() to
to ![]() .
.
– Stream ![]() passes stream
passes stream ![]() . Connect point
. Connect point ![]() to operating point
to operating point ![]() with a straight line, mark the location of
with a straight line, mark the location of ![]() .
.
– Stream ![]() is in equilibrium with stream
is in equilibrium with stream ![]() . Follow the tie-lines from stream
. Follow the tie-lines from stream ![]() to
to ![]() .
.
– Stream ![]() passes stream
passes stream ![]() . Connect
. Connect ![]() to operating point
to operating point ![]() with a straight line, mark the location of
with a straight line, mark the location of ![]() .
.
– Continue in this manner until the extract composition has reached or passed ![]() . Count the number of equilibrium stages.
. Count the number of equilibrium stages.
Watch this two-part series of videos from LearnChemE that shows how to use the Hunter Nash method to find the number of equilibrium stages required for a liquid-liquid extraction process.
- Hunter Nash Method 1: Mixing and Operating Points (9:30)
- Hunter Nash Method 2: Number of Stages (6:30)
1000 kg/hr of a feed containing 30 wt% acetone, 70 wt% water. The solvent is pure MIBK. We intend that the raffinate contain no more than 5.0 wt% acetone. How many stages will be required for each proposed solvent to feed ratio in the table below?
| target |
||||
| 1.0 | ||||
| 2.0 | ||||
| 0.2 |
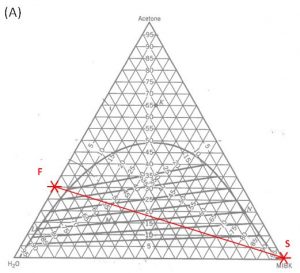
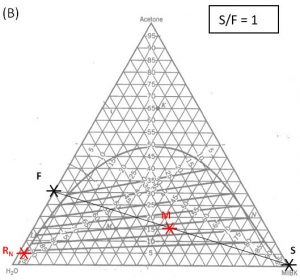
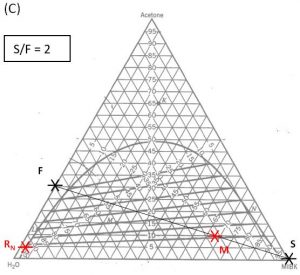
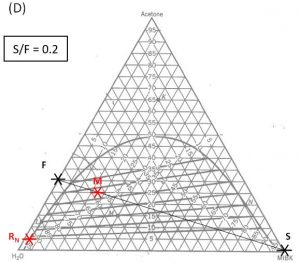

Hunter Nash Method for Finding Smin, Tank Sizing and Power Consumption for Mixer-Settler Units
Staged LLE: Hunter-Nash Method for Finding the Minimum Solvent to Feed Ratio
![]() = extract leaving stage
= extract leaving stage ![]() . This could refer to the mass of the stream or the composition of the stream.
. This could refer to the mass of the stream or the composition of the stream.
![]() = solvent entering extractor stage 1. This could refer to the mass of the stream or the composition of the stream.
= solvent entering extractor stage 1. This could refer to the mass of the stream or the composition of the stream.
![]() = generic stage number
= generic stage number
![]() = Final stage. This is where the fresh solvent
= Final stage. This is where the fresh solvent ![]() enters the system and the final raffinate
enters the system and the final raffinate ![]() leaves the system.
leaves the system.
![]() = Composition of the overall mixture. Points (
= Composition of the overall mixture. Points (![]() and
and ![]() ) and (
) and (![]() and
and ![]() ) are connected by a straight line passing through
) are connected by a straight line passing through ![]() .
.
![]() = Operating point. Every pair of passing streams must be connected by a straight line that passes through
= Operating point. Every pair of passing streams must be connected by a straight line that passes through ![]() .
.
![]() = raffinate leaving stage
= raffinate leaving stage ![]() . This could refer to the mass of the stream or the composition of the stream.
. This could refer to the mass of the stream or the composition of the stream.
![]() = solvent entering extractor stage
= solvent entering extractor stage ![]() . This could refer to the mass of the stream or the composition of the stream.
. This could refer to the mass of the stream or the composition of the stream.
![]() = mass ratio of solvent to feed
= mass ratio of solvent to feed
![]() = Minimum feasible mass ratio to achieve the desired separation, assuming the use of an infinite number of stages.
= Minimum feasible mass ratio to achieve the desired separation, assuming the use of an infinite number of stages.
![]() = Mass fraction of species
= Mass fraction of species ![]() in the raffinate leaving stage
in the raffinate leaving stage ![]()
![]() = Mass fraction of species
= Mass fraction of species ![]() in the extract leaving stage
in the extract leaving stage ![]()
![]() = Point associated with the minimum feasible
= Point associated with the minimum feasible ![]() for this feed, solvent and (raffinate or extract) composition.
for this feed, solvent and (raffinate or extract) composition. ![]() is the intersection of the line connecting points (
is the intersection of the line connecting points (![]() ,
, ![]() ) and the line that is an extension of the upper-most equilibrium tie-line.
) and the line that is an extension of the upper-most equilibrium tie-line.
Determining minimum feasible solvent mass ratio (![]() ) when (1) feed composition; (2) incoming solvent composition; and (3) outgoing raffinate composition have been specified/selected.
) when (1) feed composition; (2) incoming solvent composition; and (3) outgoing raffinate composition have been specified/selected.
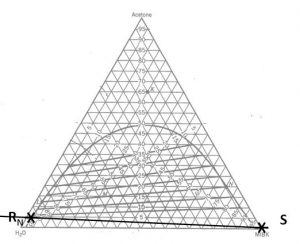
- Locate points
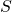 and
and 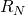 on the phase diagram. Connect with a straight line.
on the phase diagram. Connect with a straight line. - Extend the upper-most tie-line in a line that connects with the line connecting points (
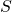 and
and 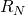 ). Label the intersection
). Label the intersection 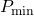 .
. - Find point
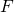 on the diagram. Draw a line from
on the diagram. Draw a line from 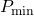 to F and extend to the other side of the equilibrium curve. Label
to F and extend to the other side of the equilibrium curve. Label 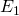 @
@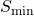 .
. - On a fresh copy of the phase diagram, label points
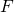 ,
, 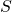 ,
, 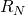 and
and 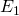 @
@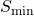 . Draw one line connecting points
. Draw one line connecting points 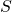 and
and 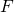 and another line connecting points
and another line connecting points 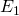 @
@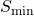
- and
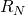 . The intersection of these two lines is mixing point
. The intersection of these two lines is mixing point 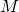 . Note the composition of species
. Note the composition of species 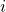 at this location.
at this location. - Calculate
(5.1) ![]()
We have a 1000 kg/hr feed that contains 30 wt% acetone and 70 wt% water. We want our raffinate to contain no more than 5.0 wt% acetone. What is the minimum mass of pure MIBK required?
Liquid-Liquid Extraction: Sizing Mixer-settler Units
![]() = volume fraction occupied by the continuous phase
= volume fraction occupied by the continuous phase
![]() = volume fraction occupied by the dispersed phase
= volume fraction occupied by the dispersed phase
![]() = viscosity of the continuous phase (mass time-1 length-1)
= viscosity of the continuous phase (mass time-1 length-1)
![]() = viscosity of the dispersed phase (mass time-1 length-1)
= viscosity of the dispersed phase (mass time-1 length-1)
![]() = viscosity of the mixture (mass time-1 length-1)
= viscosity of the mixture (mass time-1 length-1)
![]() = density of the continuous phase (mass volume-1)
= density of the continuous phase (mass volume-1)
![]() = density of the dispersed phase (mass volume-1)
= density of the dispersed phase (mass volume-1)
![]() = average density of the mixture (mass volume-1)
= average density of the mixture (mass volume-1)
![]() = impeller diameter (length)
= impeller diameter (length)
![]() = vessel diameter (length)
= vessel diameter (length)
![]() = total height of mixer unit (length)
= total height of mixer unit (length)
![]() = rate of impeller rotation (time-1)
= rate of impeller rotation (time-1)
![]() = impeller power number, read from Fig 8-36 or Perry’s 15-54 (below) based on value of
= impeller power number, read from Fig 8-36 or Perry’s 15-54 (below) based on value of ![]() (unitless)
(unitless)
![]() = Reynold’s number in the continuous phase = inertial force/viscous force (unitless)
= Reynold’s number in the continuous phase = inertial force/viscous force (unitless)
![]() = agitator power (energy time-1)
= agitator power (energy time-1)
![]() = volumetric flowrate, continuous phase (volume time-1)
= volumetric flowrate, continuous phase (volume time-1)
![]() = volumetric flowrate, dispersed phase (volume time-1)
= volumetric flowrate, dispersed phase (volume time-1)
![]() = vessel volume (volume)
= vessel volume (volume)
Tank and impeller sizing
(5.2) ![]()
Geometry of a cylinder
(5.3) ![]()
General guidelines
(5.4) ![]()
(5.5) ![]()
Impeller power consumption:
(5.6) ![]()
(5.7) ![]()
(5.8) ![]()
(5.9) ![]()
Modeling Mass Transfer in Mixer-Settler Units
![]() = density difference (absolute value) between the continuous and dispersed phases (mass volume-1)
= density difference (absolute value) between the continuous and dispersed phases (mass volume-1)
![]() = volume fraction occupied by the continuous phase
= volume fraction occupied by the continuous phase
![]() = volume fraction occupied by the dispersed phase
= volume fraction occupied by the dispersed phase
![]() = viscosity of the continuous phase (mass time-1 length-1)
= viscosity of the continuous phase (mass time-1 length-1)
![]() = viscosity of the dispersed phase (mass time-1 length-1)
= viscosity of the dispersed phase (mass time-1 length-1)
![]() = viscosity of the mixture (mass time-1 length-1)
= viscosity of the mixture (mass time-1 length-1)
![]() = density of the continuous phase (mass volume-1)
= density of the continuous phase (mass volume-1)
![]() = density of the dispersed phase (mass volume-1)
= density of the dispersed phase (mass volume-1)
![]() = average density of the mixture (mass volume-1)
= average density of the mixture (mass volume-1)
![]() = interfacial tension between the continuous and dispersed phases
= interfacial tension between the continuous and dispersed phases
(mass time-2)
![]() = interfacial area between the two phases per unit volume (area volume-1)
= interfacial area between the two phases per unit volume (area volume-1)
![]() ,
, ![]() = concentration of solute in the incoming or outgoing dispersed streams (mass volume-1)
= concentration of solute in the incoming or outgoing dispersed streams (mass volume-1)
![]() = concentration of solute in the dispersed phase if in equilibrium with the outgoing continuous phase (mass volume-1)
= concentration of solute in the dispersed phase if in equilibrium with the outgoing continuous phase (mass volume-1)
![]() = diffusivity of the solute in the continuous phase (area time-1)
= diffusivity of the solute in the continuous phase (area time-1)
![]() = diffusivity of the solute in the dispersed phase (area time-1)
= diffusivity of the solute in the dispersed phase (area time-1)
![]() = impeller diameter (length)
= impeller diameter (length)
![]() = vessel diameter (length)
= vessel diameter (length)
![]() = Sauter mean droplet diameter; actual drop size expected to range from
= Sauter mean droplet diameter; actual drop size expected to range from ![]() (length)
(length)
![]() = Murphree dispersed-phase efficiency for extraction
= Murphree dispersed-phase efficiency for extraction
![]() = gravitational constant (length time-2)
= gravitational constant (length time-2)
![]() = total height of mixer unit (length)
= total height of mixer unit (length)
![]() = mass transfer coefficient of the solute in the continuous phase (length time-1)
= mass transfer coefficient of the solute in the continuous phase (length time-1)
![]() = mass transfer coefficient of the solute in the dispersed phase (length time-1)
= mass transfer coefficient of the solute in the dispersed phase (length time-1)
![]() = overall mass transfer coefficient, given on the basis of the dispersed phase (length time-1)
= overall mass transfer coefficient, given on the basis of the dispersed phase (length time-1)
![]() = distribution coefficient of the solute,
= distribution coefficient of the solute, ![]() (unitless)
(unitless)
![]() = rate of impeller rotation (time-1)
= rate of impeller rotation (time-1)
![]() = Eotvos number = gravitational force/surface tension force (unitless)
= Eotvos number = gravitational force/surface tension force (unitless)
![]() = Froude number in the continuous phase = inertial force/gravitational force (unitless)
= Froude number in the continuous phase = inertial force/gravitational force (unitless)
![]() = minimum impeller rotation rate required for complete dispersion of one liquid into another
= minimum impeller rotation rate required for complete dispersion of one liquid into another
![]() = Reynold’s number in the continuous phase = inertial force/viscous force (unitless)
= Reynold’s number in the continuous phase = inertial force/viscous force (unitless)
![]() = Sherwood number in the continuous phase = mass transfer rate/diffusion rate (unitless)
= Sherwood number in the continuous phase = mass transfer rate/diffusion rate (unitless)
![]() = Schmidt number in the continuous phase = momentum/mass diffusivity (unitless)
= Schmidt number in the continuous phase = momentum/mass diffusivity (unitless)
![]() = Weber number = inertial force/surface tension (unitless)
= Weber number = inertial force/surface tension (unitless)
![]() = volumetric flowrate of the dispersed phase (volume time-1)
= volumetric flowrate of the dispersed phase (volume time-1)
![]() = vessel volume (volume)
= vessel volume (volume)
Calculating ![]()
(6.1) ![]()
(6.2) ![]()
(6.3) ![]()
Estimating Murphree efficiency for a proposed design
Sauter mean diameter
(6.4) ![]()
(6.5) ![]()
(6.6) ![]()
mass transfer coefficient of the solute in each phase
(6.7) ![]()
(6.8) ![]()
(6.9) 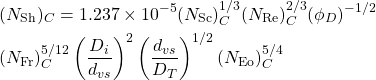
(6.10) ![]()
(6.11) ![]()
(6.12) ![]()
(6.13) ![]()
Overall mass transfer coefficient for the solute
(6.14) ![]()
Murphree efficiency
(6.15) ![]()
(6.16) ![]()
Experimental assessment of efficiency
(6.17) ![]()
1000 kg/hr of 30 wt% acetone and 70 wt% water is to be extracted with 1000 kg/hr of pure MIBK. Assume that the extract is the continuous phase, a residence time of 5 minutes in the mixing vessel, standard sizing of the mixing vessel and impeller. Find the power consumption and Murphree efficiency if the system operates at ![]() , controlled at the level of 1 rev/s. Ignore the contribution of the solute and the co-solvent to the physical properties of each phase.
, controlled at the level of 1 rev/s. Ignore the contribution of the solute and the co-solvent to the physical properties of each phase.
- MIBK
- density = 802 kg m-3
- viscosity = 0.58 cP
- diffusivity with acetone at 25°C = 2.90×10-9 m2 s-1
- Water
- density = 1000 kg m-3
- viscosity = 0.895 cP
- diffusivity with acetone at 25°C = 1.16×10-9 m2 s-1
- The interfacial tension of water and MIBK at 25°C = 0.0157 kg s-2. Use the ternary phase diagram to find
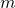 .
.
Liquid-Liquid Extraction Columns
![]() = density difference (absolute value) between the continuous and dispersed phases (mass volume-1)
= density difference (absolute value) between the continuous and dispersed phases (mass volume-1)
![]() = viscosity of the continuous phase (mass time-1 length-1)
= viscosity of the continuous phase (mass time-1 length-1)
![]() = density of the continuous phase (mass volume-1)
= density of the continuous phase (mass volume-1)
![]() = density of the dispersed phase (mass volume-1)
= density of the dispersed phase (mass volume-1)
![]() = interfacial tension between the continuous and dispersed phases
= interfacial tension between the continuous and dispersed phases
(mass time-2)
![]() = column diameter (length)
= column diameter (length)
![]() = total height of column (length)
= total height of column (length)
![]() = height of equilibrium transfer stage (length)
= height of equilibrium transfer stage (length)
![]() = mass flowrate of the entering continuous phase (mass time-1)
= mass flowrate of the entering continuous phase (mass time-1)
![]() = mass flowrate of the entering dispersed phase (mass time-1)
= mass flowrate of the entering dispersed phase (mass time-1)
![]() = required number of equilibrium stages
= required number of equilibrium stages
![]() = characteristic rise velocity of a droplet of the dispersed phase (length time-1)
= characteristic rise velocity of a droplet of the dispersed phase (length time-1)
![]() = superficial velocity of phase
= superficial velocity of phase ![]() (C = continuous, downward; D = dispersed, upward) (length time-1)
(C = continuous, downward; D = dispersed, upward) (length time-1)
![]() = volumetric flowrate of phase
= volumetric flowrate of phase ![]() (volume time-1)
(volume time-1)
(7.1) ![]()
definition of superficial velocity
(7.2) ![]()
(7.3) ![]()
for operation at 50% of flooding
(7.4) ![]()
for rotating-disk columns, ![]() = 8 to 42 inches, with one aqueous phase
= 8 to 42 inches, with one aqueous phase
(7.5) ![]()
(7.6) ![]()
1000 kg/hr of 30 wt% acetone and 70 wt% water is to be extracted with 1000 kg/hr of pure MIBK in a 2-stage column process. Assume that the extract is the dispersed phase. Ignoring the contribution of the solute and the co-solvent to the physical properties of each phase, find the required column diameter and height.
- MIBK
- density = 802 kg m-3
- viscosity = 0.58 cP
- Water
- density = 1000 kg m-3
- viscosity = 0.895 cP
- The interfacial tension of water and MIBK at 25°C = 0.0157 kg s-2.

