2 Mass Transfer in Gas-liquid Systems
In chemical separations, we can use thermodynamic models to predict the composition in each phase at equilibrium. For example, Raoult’s Law describes the compositions of vapor and liquid phases at equilibrium. Mass transfer models help us understand how we can manipulate the process to reach equilibrium in a faster or more economical manner.
This chapter will briefly review fundamentals of mass transfer in gas-liquid systems because many of the separation processes in this handbook involve the movement of species between gas and liquid phases.
Diffusion-based Mass Transfer
![]() = molar flux of
= molar flux of ![]() relative to the molar-average velocity of the mixture in the
relative to the molar-average velocity of the mixture in the ![]() direction
direction
![]() = mutual diffusion coefficient of
= mutual diffusion coefficient of ![]() in
in ![]() (
(![]() )
)
![]() = molar concentration of
= molar concentration of ![]()
![]() = molar flux of species
= molar flux of species ![]()
![]() = total molar flux
= total molar flux
![]() = mole fraction of species
= mole fraction of species ![]()
![]() = total molar concentration
= total molar concentration
(1.1) ![]()
(1.2) ![]()
Falling Liquid Film with Gaseous Solute A Diffusing into Liquid B
![]() = liquid flow rate per unit width of film
= liquid flow rate per unit width of film ![]() (mass length
(mass length![]() time
time![]() )
)
![]() = film thickness (length)
= film thickness (length)
![]() = metric used in selecting the appropriate equation for calculating
= metric used in selecting the appropriate equation for calculating ![]()
![]() = dynamic viscosity of the liquid (pressure time)
= dynamic viscosity of the liquid (pressure time)
![]() = density of the liquid (mass volume-1)
= density of the liquid (mass volume-1)
![]() = available area for mass transfer =
= available area for mass transfer = ![]() (length2)
(length2)
![]() = concentration of species
= concentration of species ![]() in liquid
in liquid ![]() (mol volume-1)
(mol volume-1)
![]() = concentration of species
= concentration of species ![]() in liquid
in liquid ![]() at the gas/liquid interface (mol volume
at the gas/liquid interface (mol volume![]() )
)
![]() = concentration of species
= concentration of species ![]() in liquid
in liquid ![]() when the liquid enters the enters (mol volume
when the liquid enters the enters (mol volume![]() )
)
![]() = log mean concentration difference driving force (mol volume
= log mean concentration difference driving force (mol volume![]() )
)
![]() = bulk concentration of species
= bulk concentration of species ![]() in liquid
in liquid ![]() at any position
at any position ![]() (mol volume
(mol volume![]() )
)
![]() = bulk concentration of species
= bulk concentration of species ![]() in liquid
in liquid ![]() at any position
at any position ![]() (mol volume
(mol volume![]() )
)
![]() = diffusivity of solution
= diffusivity of solution ![]() in liquid
in liquid ![]() (length
(length![]() time
time![]() )
)
![]() = gravitational constant (length time
= gravitational constant (length time![]() )
)
![]() = Henry’s Law constant for solute
= Henry’s Law constant for solute ![]() in our liquid at our system temperature (volume pressure mol
in our liquid at our system temperature (volume pressure mol![]() )
)
![]() = average mass transfer coefficient (length time
= average mass transfer coefficient (length time![]() )
)
![]() = film height (length)
= film height (length)
![]() = molar flow rate of species
= molar flow rate of species ![]() (mol time
(mol time![]() )
)
![]() = Reynolds number (dimensionless)
= Reynolds number (dimensionless)
![]() = Schmidt number (dimensionless)
= Schmidt number (dimensionless)
![]() = Peclet number for mass transfer (dimensionless)
= Peclet number for mass transfer (dimensionless)
![]() = partial pressure of species
= partial pressure of species ![]() in the gas phase (pressure)
in the gas phase (pressure)
![]() = flow cross section per wetted perimeter (length)
= flow cross section per wetted perimeter (length)
![]() = bulk velocity of the falling film in the
= bulk velocity of the falling film in the ![]() direction (length time
direction (length time![]() )
)
![]() = film width (length)
= film width (length)
Reynolds number for a falling film
(1.3) ![]()
Rate
(1.4) ![]()
(1.5) ![]()
Film thickness
(1.6) ![]()
Hydraulic radius for a falling film Flow cross section: ![]() ; wetted perimeter:
; wetted perimeter: ![]()
(1.7) ![]()
Schmidt number
(1.8) ![]()
Peclet number for mass transfer
(1.9) ![]()
Mass transfer coefficient ![]()
(1.10) ![]()
if ![]() < 0.001,
< 0.001,
(1.11) ![]()
if ![]() > 0.1,
> 0.1,
(1.12) ![]()
if ![]() > 1,
> 1,
(1.13) ![]()
Henry’s Law, applies at the interface
(1.14) ![]()
Log mean concentration difference
(1.15) ![]()
Example
10 mL/s of water at 25![]() C flows down a wall that is 1.0 m wide and 3.0 m high. This film is in contact with pure CO2 at 1.0 atm, 25
C flows down a wall that is 1.0 m wide and 3.0 m high. This film is in contact with pure CO2 at 1.0 atm, 25![]() C. Find the rate of absorption of CO2 into the water (kmol/s).
C. Find the rate of absorption of CO2 into the water (kmol/s).
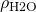 = 998 kg/m3
= 998 kg/m3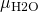 = 8.90×10-4 Pa s
= 8.90×10-4 Pa s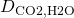 = 1.96×10-9 m2/s
= 1.96×10-9 m2/s-
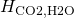 = 29.41 L atm mol-1
= 29.41 L atm mol-1
Velocity and Concentration Boundary Layers for Laminar Horizontal Flow Across a Flat Plate
![]() = thickness (height) of the velocity boundary layer (length)
= thickness (height) of the velocity boundary layer (length)
![]() = thickness (height) of the concentration boundary layer (length)
= thickness (height) of the concentration boundary layer (length)
![]() = fluid viscosity (pressure time) or (mass length-1 time-1)
= fluid viscosity (pressure time) or (mass length-1 time-1)
![]() = fluid density (mass volume-1)
= fluid density (mass volume-1)
![]() = concentration of species A in fluid B at some point (
= concentration of species A in fluid B at some point (![]() ,
, ![]() ) (mol volume-1)
) (mol volume-1)
![]() = initial concentration of species A in fluid B (mol volume-1)
= initial concentration of species A in fluid B (mol volume-1)
![]() = concentration of species A in fluid B at the interface (mol volume-1)
= concentration of species A in fluid B at the interface (mol volume-1)
![]() = diffusivity of solute A in fluid B (length2 time-1)
= diffusivity of solute A in fluid B (length2 time-1)
![]() = Reynolds number (dimensionless)
= Reynolds number (dimensionless)
![]() = Schmidt number (dimensionless)
= Schmidt number (dimensionless)
![]() = free-stream velocity of the fluid in the
= free-stream velocity of the fluid in the ![]() direction (length time-1)
direction (length time-1)
![]() = fluid velocity in the x direction at some point (
= fluid velocity in the x direction at some point (![]() ,
, ![]() ) (length time-1)
) (length time-1)
![]() = horizontal distance from the leading edge of the flat plate (length)
= horizontal distance from the leading edge of the flat plate (length)
![]() = vertical distance from the plate surface (length)
= vertical distance from the plate surface (length)
(2.1) ![]()
(2.2) ![]()
(2.3) ![]()
(2.4) ![]()
(2.5) ![]()
(2.6) ![]()
Example
Air at 100![]() C and 1.0 atm with a free-stream velocity of 5.0 m/s flows over a 3.0 m long flat plate made of naphthalene.
C and 1.0 atm with a free-stream velocity of 5.0 m/s flows over a 3.0 m long flat plate made of naphthalene.
(a) At what horizontal position does flow become turbulent?
(b) What is the thickness of the velocity boundary layer at that point?
(c) What is the thickness of the concentration boundary layer at that point?
(d) What is the concentration of naphthalene in the air at this horizontal position and vertical position that is half of the height of the concentration boundary layer?
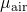 = 2.15×10-5 kg m-1 s-1
= 2.15×10-5 kg m-1 s-1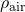 = 0.0327 kmol m-3
= 0.0327 kmol m-3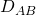 = 0.94×10-5 m2 s-1
= 0.94×10-5 m2 s-1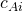 = 4.3×10-3 kmol m-3
= 4.3×10-3 kmol m-3
Film Theory and Overall Mass Transfer Coefficients
![]() = thickness of the film in which
= thickness of the film in which ![]() (length)
(length)
![]() = total concentration of liquid B (mol volume-1)
= total concentration of liquid B (mol volume-1)
![]() = concentration of species A in liquid B at the interface (mol volume-1)
= concentration of species A in liquid B at the interface (mol volume-1)
![]() = bulk concentration of species A in liquid B (mol volume-1)
= bulk concentration of species A in liquid B (mol volume-1)
![]() = concentration of species A in liquid B at equilibrium with the bulk gas phase (mol volume-1)
= concentration of species A in liquid B at equilibrium with the bulk gas phase (mol volume-1)
![]() = diffusivity of species A in liquid B (area time-1)
= diffusivity of species A in liquid B (area time-1)
![]() = Henry’s Law constant for equation of the form
= Henry’s Law constant for equation of the form ![]() ;
;
(mol volume-1 pressure-1)
![]() = liquid-phase mass transfer coefficient, with respect to concentration driving force (length time-1)
= liquid-phase mass transfer coefficient, with respect to concentration driving force (length time-1)
![]() = overall mass transfer coefficient, with respect to pressure driving force (mol time-1 area-1 pressure-1)
= overall mass transfer coefficient, with respect to pressure driving force (mol time-1 area-1 pressure-1)
![]() = overall mass transfer coefficient, with respect to concentration driving force (length time-1)
= overall mass transfer coefficient, with respect to concentration driving force (length time-1)
![]() = gas-phase mass transfer coefficient, pressure driving force (mol time-1 area-1 pressure-1)
= gas-phase mass transfer coefficient, pressure driving force (mol time-1 area-1 pressure-1)
![]() = molar flux (mol area-1 time-1)
= molar flux (mol area-1 time-1)
![]() = partial pressure of species A in the bulk gaseous phase (pressure)
= partial pressure of species A in the bulk gaseous phase (pressure)
![]() = partial pressure of species A in a gas at equilibrium with the bulk liquid phase (pressure)
= partial pressure of species A in a gas at equilibrium with the bulk liquid phase (pressure) ![]() = mole fraction of species A in liquid B at the interface
= mole fraction of species A in liquid B at the interface
![]() = bulk mole fraction of species A in liquid B
= bulk mole fraction of species A in liquid B
Film Theory
(3.1) ![]()
Example
SO2 is absorbed from air into water using a packed absorption tower. At a specific location in the tower, we know that the pressure of SO2 is 0.15 atm. Measurements of the gas composition above and below this location in the tower have told us that the flux of SO2 into the water is 0.0270 kmol SO2 m-2 hr-1. We were able to sample the bulk liquid phase at this location and found that it contained 3.0×10-4 mol SO2/mol. Assuming that this system fits film theory, find the thickness of the film.
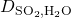 = 1.7*10-9 m2 s-1
= 1.7*10-9 m2 s-1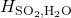 = 1.2 L atm mol-1
= 1.2 L atm mol-1
Two-Film Theory, Overall Mass Transfer Coefficients, Gas/Liquid
(3.2) ![]()
(3.3) ![]()
(3.4) ![]()
(3.5) ![]()
(3.6) ![]()
(3.7) ![]()
Example
We intend to use water to absorb SO2 from air. The incoming air is at 50°C and 2.0 atm and contains 0.085 mol SO2/mol. The incoming water, also at 50°C, already contains 0.0010 mol SO2/mol.
(a) Which phase is most limiting to mass transfer?
(b) What is the expected initial flux value?
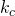 = 0.18 m hr-1
= 0.18 m hr-1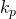 = 0.040 kmol hr-1 m-2 kPa-1
= 0.040 kmol hr-1 m-2 kPa-1 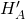 (50°C) = 0.76 mol atm-1 L-1
(50°C) = 0.76 mol atm-1 L-1

